Math Arguments

Medieval Ballistics

Tartaglia




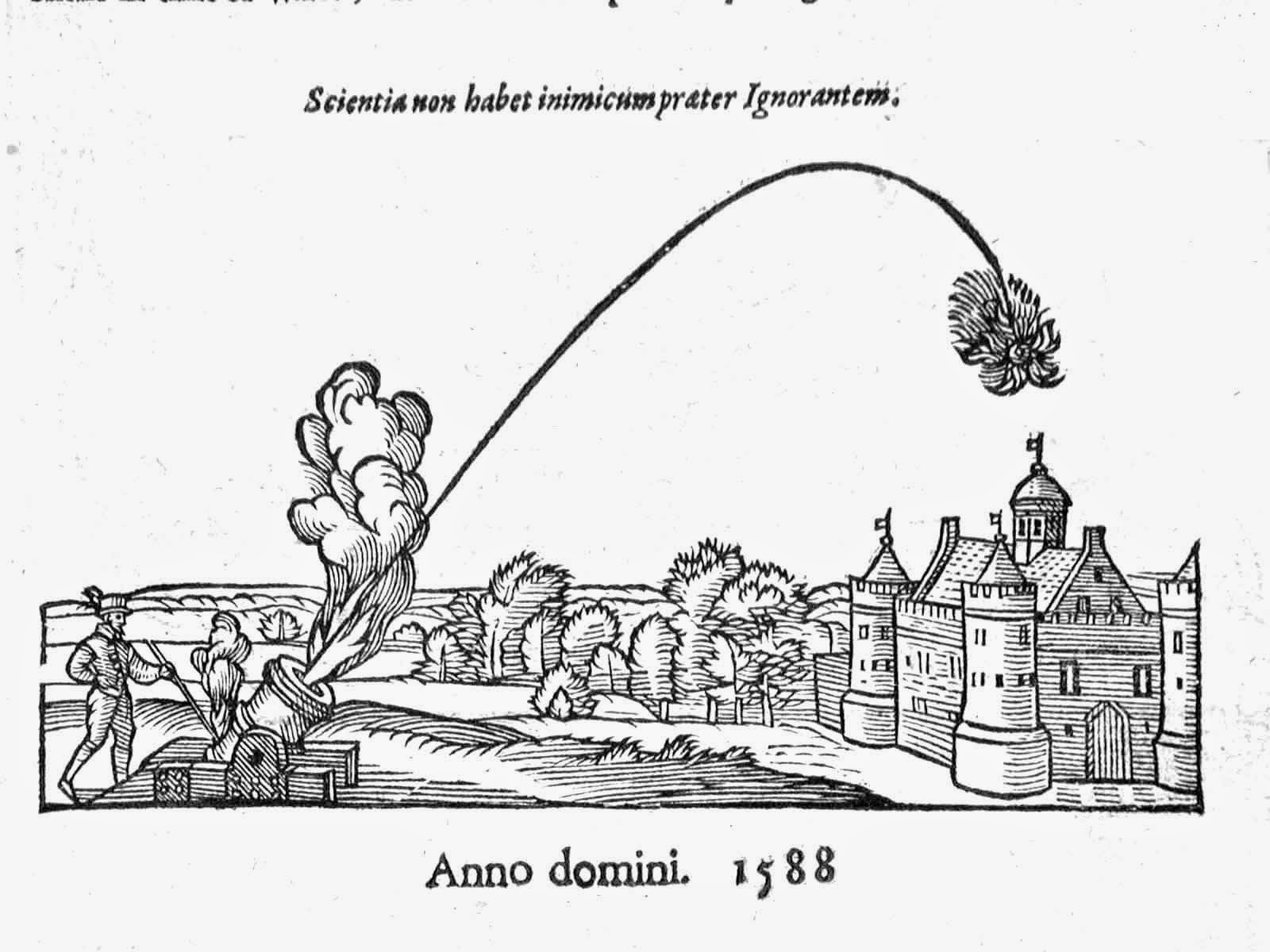
Using the angle between line of sight to the projectile and the horizontal, show why the trajectory was considered to be in this shape for many centuries.

(Hint: consider that only those viewing from BEHIND could see the projectile. UPDATE: Yes, those in front could see something coming. "I couldn't figure out what it was coming closer and closer ... then it hit me.")




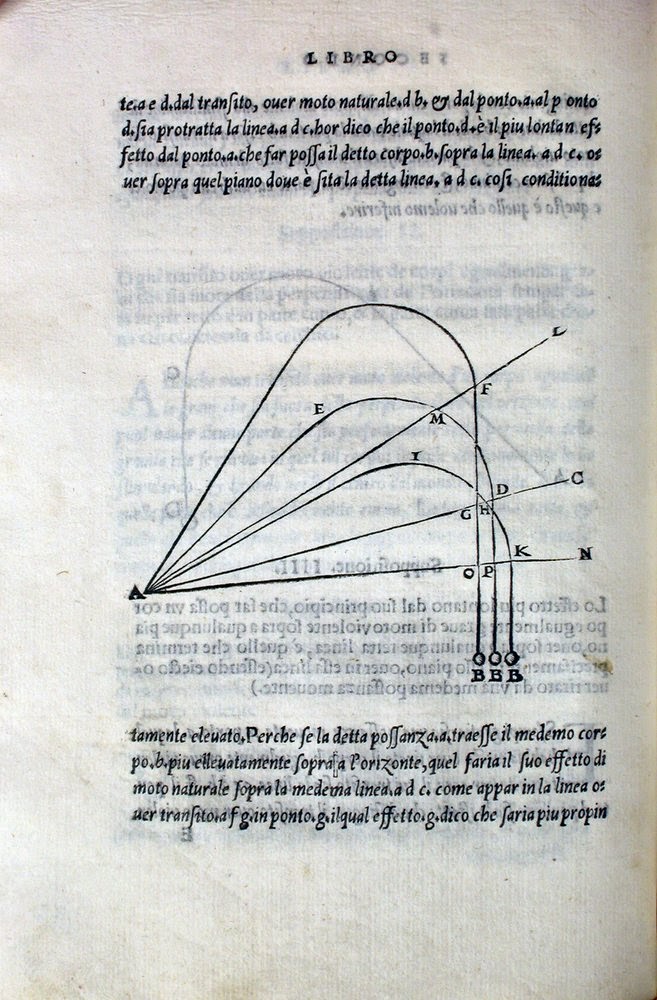
This may be more than you want ... if so, pay no attention to the following instructions.

In DESMOS or Geogebra, graph the parabolic arc. We'll assume for simplicity that the cannonball begins at \( (0,0) \) and hits at \( (1000,0) \) with max at \( (500,250) \).

An exercise for Algebra 2: create the parabola that fits those three points.

\( y=x(x-1000)/1000) \).

Place \(A\) at \((-10,0)\) and \(B\), a point on the function \( f(x) \). Place \( C \) at \( (500,0) \) so we have three points.

Create line segments \( \overline{\rm AB} \) and \( \overline{\rm AC} \). Measure \(\angle CAB\). Drag \(B\) along the curve, paying attention to the angle.  What do you see?

How does it change as you move \(B\)?
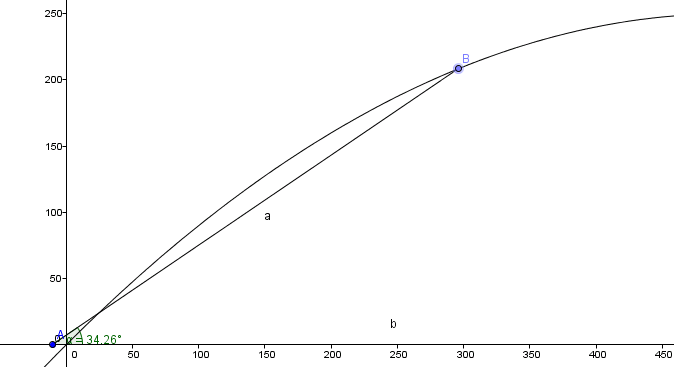