12 of 15 Items .... Type: Pedagogy
Problems, Questions, and Puzzles to spark discussion and argument in the maths classroom.
Navigation:
- by Source
- by Course
.... - Problem Type: 101 Things
3ACT
ASN
ChatGPT
Combinatorics
Comparisons
Constructions
Create a Problem
DebateMath
Definitions
DESMOS Art
Desmos Challenge
DoThis
Epiphany
Equity
Explainer
Find the Error
Find the Pattern
Game
Geometry Snacks
Graphicacy
Graphical Approach
How Many Ways?
How Many?
Illusion
Impossible Problem
MathArt
MathStrategy
MathTip
Meme
Mimizu
Modeling
New
New Understanding
Notation
Notice, Wonder
Number Theory
Number_Search
Number_Talks
Open Middle
Optimization
Pedagogy
Policy
Proof Without Words
Puzzle
Rates
Ratios & Proportions
Raw Pure Math
Regressions
Sensible?
Sidewalk Chalk
Smart Substitution
Starters
Strategy
Terminology
The Hook
The RealWorld
Topology
Understandings
VennWords
Wait. Really?
WCYDWT
What If
What is This?
What Questions
Which Would You Choose?
WODB
Yohaku
. . . View This Fullsize
Here are 8 circle theorems, written by a Brit so you'll have to "translate" to American English ...
Are these the most important ones?
Are there any that are missing that you feel should be included in this group?
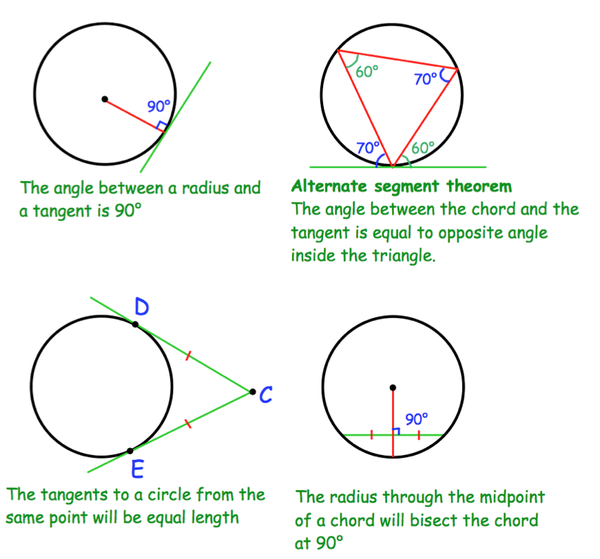

.: [GEOM], [Corbettmaths], [Pedagogy].
. . . View This Fullsize
Here are some questions (to teach precalc & stats):
What's a logarithm?
What's a situation in which mean & median are different & one might be preferable?
How do you see math & social justice combining?
What's your fave math thing?
Then: minilesson on what Ss are learning. I think a minilesson is good & especially if it's on the material they'd be broaching. More interested in familiarity with content & pedagogical moves than a pre-polished lesson.
I also asked about math/gender since I teach at a girls school.
Views/whys on tracking also good.
.: [Teacher Education], [Benjamin Dickman], [Pedagogy].
. . . View This Fullsize
1) What is math?
2) What's an interesting math problem you've recently solved?
3) How can you tell if the structure (rather than content) of a lesson you've taught was effective?
.: [Teacher Education], [internet], [Pedagogy].
. . . View This Fullsize
From our recent group True-False quiz:
Two of these had correct response rates of 90%, the other two 60%. Which are which?

.: [Teacher Education], [Frank Noschese], [Pedagogy].
. . . View This Fullsize
What's your take here? Does it matter if the tape diagram attempts to represent the size of the relationships? Does it matter if it's the student's or the teacher's model?
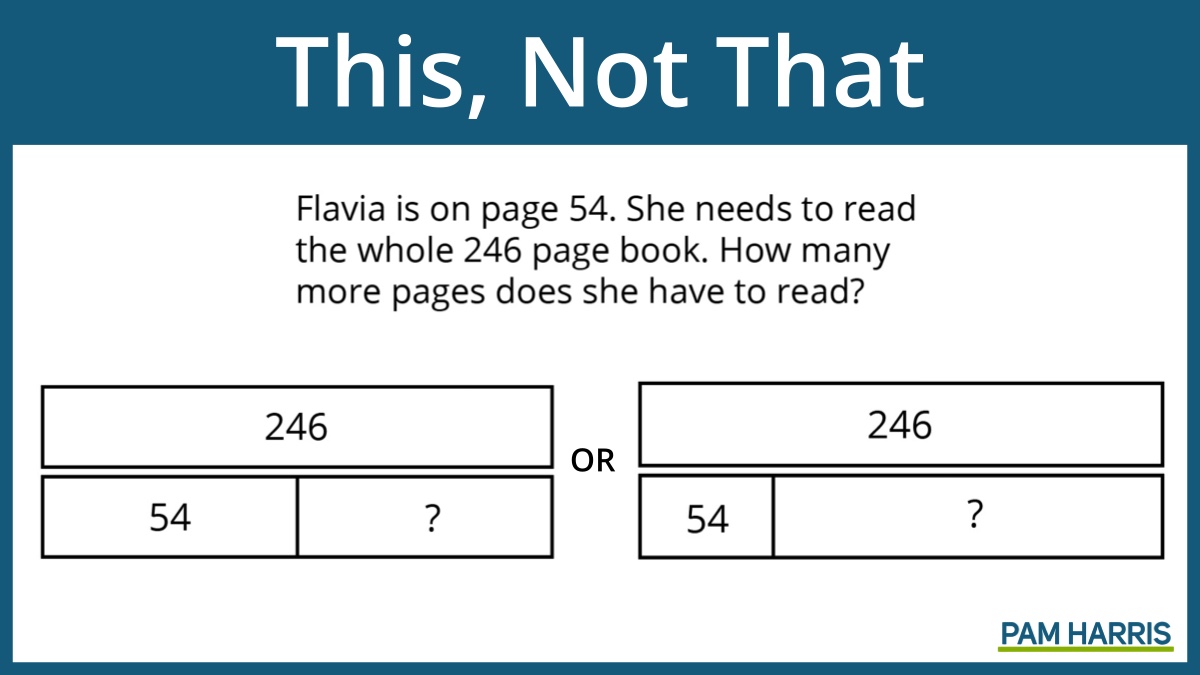
I Personally think that diagrams should be fairly true, or be labelled as "Not to Scale" with explicit instructions to consider re-drawing the diagram in any such case.
.: [Teacher Education], [Pam Harris], [Pedagogy].
. . . View This Fullsize
What do you think of this suggestion?
Should we stick to Algebra 1, Geometry, Algebra 2, Pre-Calculus, and keep calling things Remedial, College Prep, Honors?
"This gets us started on another large conversation. In my dream there would be three ways that students could take math in high school.
1. Math for future scientists and engineers.
2. Math for daily life.
3. Math for fun, beauty, and intellectual appreciation."
~ Steven Strogatz
.: [ALL], [Steven Strogatz], [Pedagogy].
. . . View This Fullsize
Periodically, the conversation turns to Calculus.
"Why is Calculus the goal, the high point, of every HS math sequence for every student?"
.: [ALL], [T.R.Milne], [Pedagogy].
. . . View This Fullsize
In the 1960's and 1970's, the United States was trying to "catch up" to Russian education standards. Every student was told that in order to graduate, they had to take (and pass) Algebra 1, Geometry, and Algebra 2?
Does that make sense?
I maintain that Statistical Reasoning should be part of that minimum, and that Algebra 2 does not have to be?
What do you think?
.: [ALL], [T.R.Milne], [Pedagogy].
. . . View This Fullsize
What do you think of this method?
"I write a lot of technical and narrative documents at work, most 2 to 6 pages. I wanted to share an editing technique that a past mentor taught me that I think is useful and under-valued for these kinds of documents: counting.~Marc Brooker
Start off with a list of key points you want the document to communicate. In two pages, this probably should be between one and three. In six pages, maybe you can pull off four. In a twelve page research paper, maybe a couple more, but that's it.
Then rank your points in order of how important it is that the audience understand them. Think about how complex, controversial, or unfamiliar each point is. Look at this from the reader's perspective, not yours.
Next, go through your document sentence by sentence and count the words you dedicate to each point. Compare the two lists. Are you spending your word budget in approximate order of importance? Does it reflect the order of complexity?
If it's far off, and you've spent a lot of words saying something unimportant or easy to understand, then you've got a great place to start editing. If you've spent space on things that aren't your core points, that's an even better editing opportunity.
Next, look at the order of how you spend time on each point. Does that order reflect the order of importance? Does it tell a story that either leads with, or leads to, your most important points?
"Extracting the outline" in this way, you get to step back from your own writing, and make sure you haven't lost your core points in a sea of details. It's not the last word in editing, but I do it every time I write an important document I really need the audience to understand."
29 Apr 2021
.: [Language], [internet], [Pedagogy].
. . . View This Fullsize
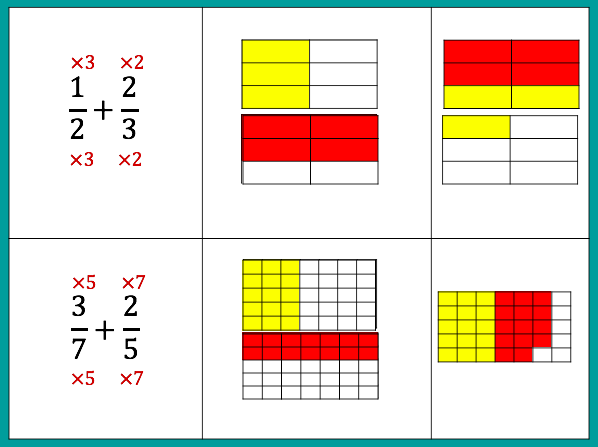
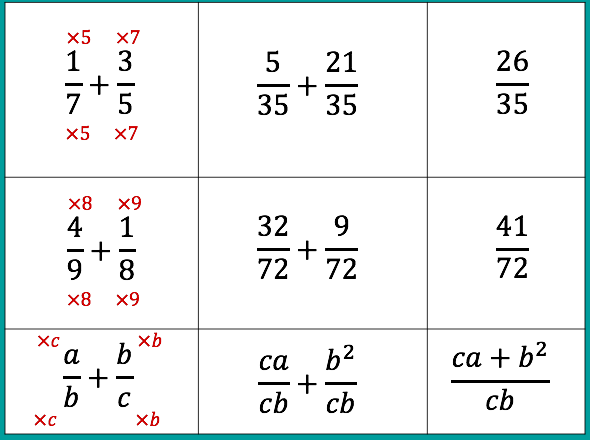
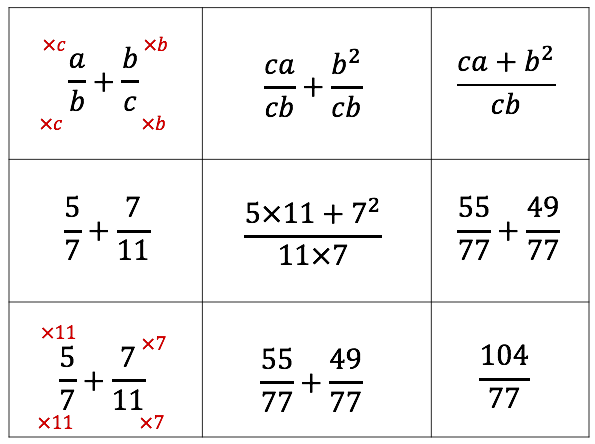
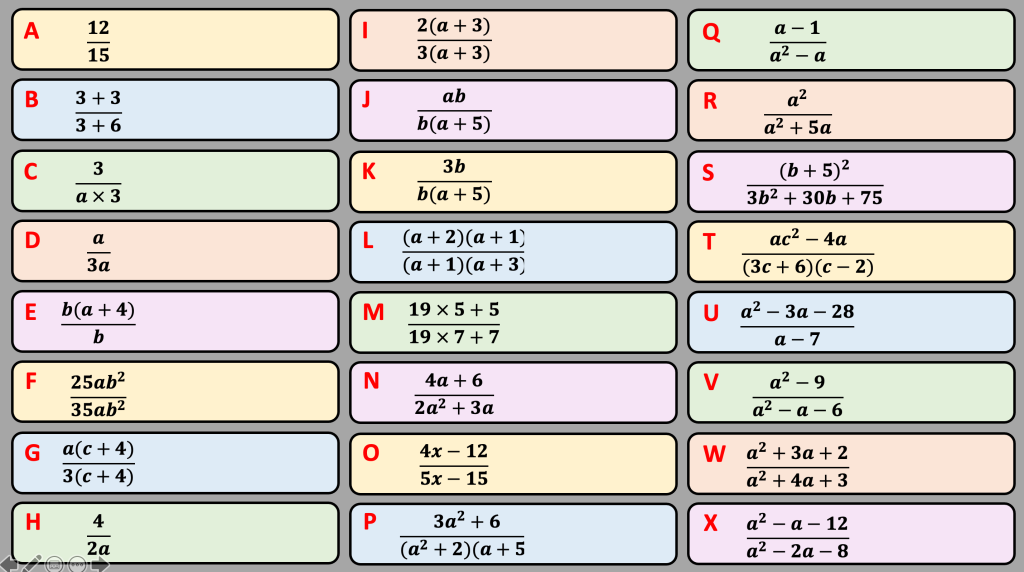
At what grade level, and during which topic (or after which topic) can we reasonably expect that a student can solve this without a calculator?

.: [Teacher Education], [SKG], [Pedagogy].
. . . View This Fullsize
At what grade level, and during which topic (or after which topic) can we reasonably expect that a student can solve this without a calculator?
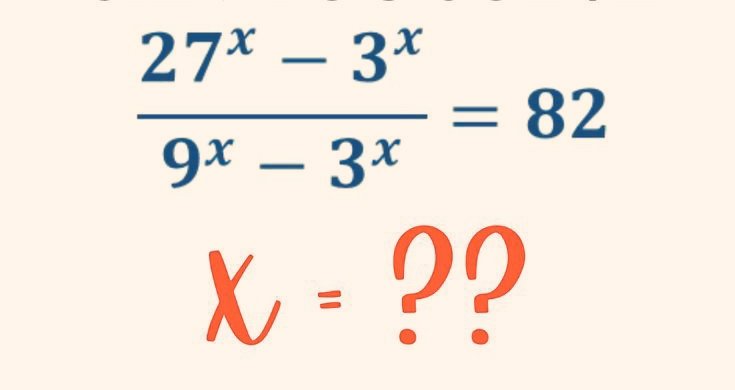
\( \dfrac{27^x-3^x}{9^x-3^x} \)
.: [Teacher Education], [SKG], [Pedagogy].